Beauty comes in many shapes

Mathematics and physics are closely connected disciplines. Throughout the history of science this connection has proven fruitful, and in many cases surprising. How can it be that abstract mathematical equations and relations are so successful in describing or predicting, time and again, physical effects that we encounter in the lab or in everyday life? The physicist Eugene Wigner (1902–1995) called this phenomenon the “unreasonable effectiveness of mathematics in the natural sciences” [1]. A case in point is the area of topology. Topology is a branch of geometry that is concerned, roughly speaking, with describing how the shape of an object can be changed by bending and stretching it. Tools and insights from this abstract field have been used for ‘practical’ purposes since its very beginning, and they still are. Indeed, one of the seminal works in the field was based on a concrete problem, when the Swiss polymath Leonhard Euler (1707–1783) solved the famous Seven Bridges of Königsberg problem — answering the question whether one can walk through the city of Königsberg, with its two large islands and historically seven bridges, and cross each of the bridges exactly once. One cannot. To reach that conclusion, Euler used methods that became later a part of the foundations for the field of topology.

Following new patterns

The articles of Huber [2] and of Zilberberg (together with Yaacov Kraus of the Holon Institute of Technology, Israel; [3]) are concerned with how topology is used today to explain unusual physical properties of materials — and how concepts of topology can be harnessed to predict novel properties of materials that, in turn, can be used in practical applications. In physics, effects rooted in geometry have long been known. These effects relate in many cases to the sort of patterns in which large ensemble of small entities (rather than roads and bridges) can be arranged. Take water molecules, for example. We normally encounter ensembles of water molecules in three forms: as gaseous vapour, where the molecules move largely independent of one another; as liquid water, where the molecules interact and perform concerted movements; or as solid ice, where the molecules are frozen into fixed positions. The organizing principles underlying these three distinctly different forms of water, and how to transform one into another, are well understood. The same basic rules apply in many materials that change their properties when, typically, the temperature is changed.

But there are patterns and transformations that cannot be explained in this classical framework of physics. And here is where topology comes into play. An important recent example of where advanced ‘topological properties’ are needed to understand the behaviour of a class of materials is that of topological insulators. These materials have the counterintuitive property that they are electrical insulators in the inside — that is, no electrical current can flow there — but the materials conduct electricity on the surface. The prediction of the existence of topological insulators and the subsequent experimental verifications have given the field of ‘topological matter’ an enormous push, with the promise of interesting technological applications in electronics.

From quantum physics to acoustics

Topological effects are traditionally explored in the context of quantum physics. But they can also be observed, and exploited, in mechanical systems, as Huber describes in his article [2]. The differences in the systems studied in classical mechanics and in quantum mechanics are enormous — swinging pendula and spinning gyroscopes here, atoms and electrons there. The mathematics to describe the two worlds, however, can be quite similar. That such similarities exist has been well known. But that the similarities are so deep that insights gained in the field of topological quantum systems can be transferred to mechanical systems, that has come as a surprise to many.

Beyond exploring mathematical similarities, practical mechanical systems in which interesting topological patterns can be created have been constructed in recent years, and Huber’s group has made important contributions to this field of ‘topological mechanics’. Last year, Huber and his student Roman Süsstrunk presented a mechanical analogue to topological insulators [4]. They showed theoretically, and demonstrated experimentally, that the phenomenon of having ‘something moving’ at the surface while motion is inhibited inside the material can be observed in an array of pendula coupled to each other (see figure above). Together with related works by others [2], a new area of research has been created that is now systematically explored [5]. And as is the case with topological insulators for electrons, there is the prospect for practical applications for mechanical topological systems. In particular, Huber expects that the topological properties of such materials could lead to novel approaches in acoustics, for example for sound isolation.

On to higher dimensions

In their separate article [3], Zilberberg and Kraus explore a connection of topology to physical systems that, in themselves, are things of marvel: quasicrystals. The building blocks of ‘normal’ crystals are arranged in a periodical — that is, in a strictly repeating — pattern. In quasicrystals, in contrast, the building blocks are repeated in a less regular fashion (see figure below). Quasicrystals were discovered in 1981 by Dan Shechtman. His discovery spurred a debate that lasted for many years, questioning whether these materials, which are so different from any other crystals that were known until then, are ‘real’. Quasicrystals were proven to be real indeed, and their existence led to a new definition of what a crystal is. Shechtman was awarded the Nobel Prize in Chemistry for his discovery in 2011.

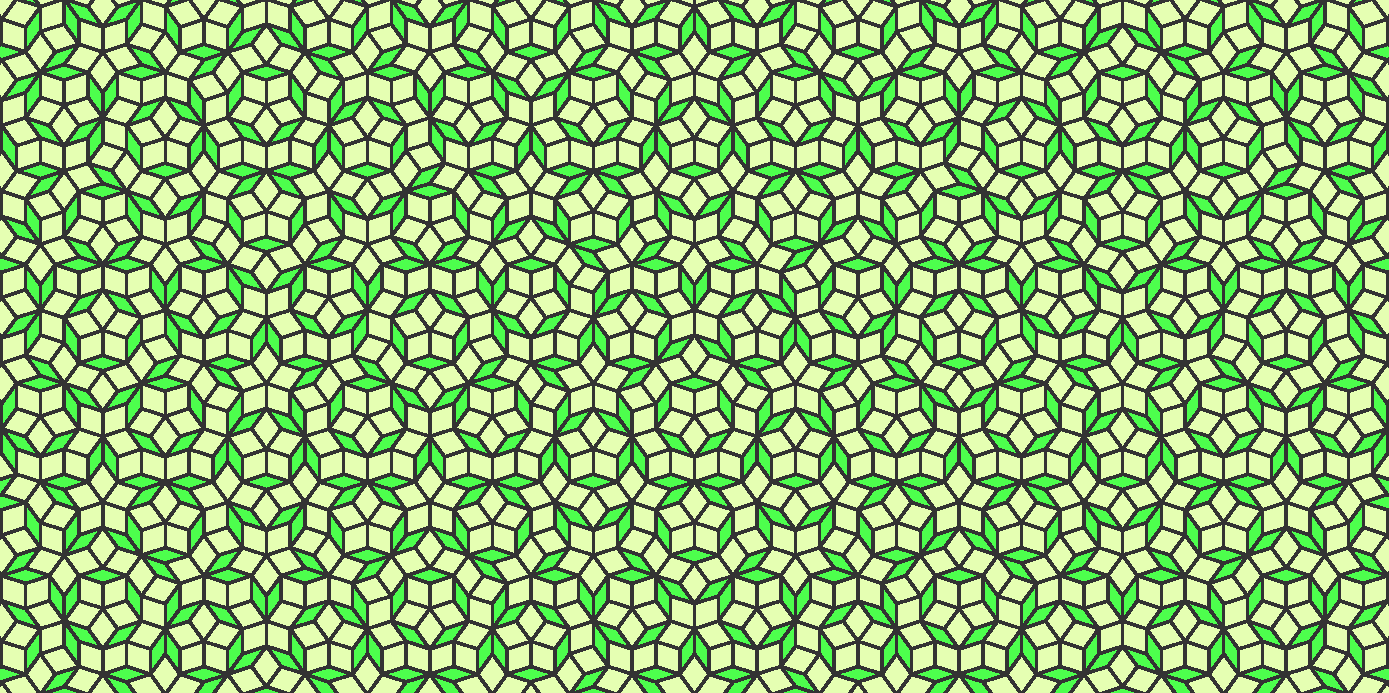
In the same year as Shechtman’s award, Kraus and Zilberberg (then both at the Weizmann Institute of Science, Israel) found together with co-workers surprising connections between quasicrystals and topological insulators (published in 2012; [6]). Theoretically and in experiments, the team established that materials with quasicrystalline order not only act analogous to a topological insulator, but they also showed that the mathematical description of this phenomenon leads to ‘higher dimensions’. More specifically, the behaviour of a one-dimensional quasicrystal, for example, is appropriately described by the mathematics of a two-dimensional space. Zilberberg and Kraus explain [3] that this connection between dimensions provides an intriguing route towards exploring experimentally mathematical models that cannot be easily accessed otherwise, such as four-dimensional topological insulators [7]. On the other hand, a deeper understanding of topological properties of quasicrystals should also help in developing applications based on these materials.

A diverse field, with a rich history at ETH Zurich

The commentaries of Huber [2] and Zilberberg and Kraus [3] in Nature Physics form parts of a broader collection of articles on ‘topological matter’, written by leading international experts and highlighting emerging directions in the field. Researchers at ETH are currently making substantial contributions to several areas in which connections between topology and physics are central — topological effect in mechanical systems and quasicrystals are merely two of them. Over the past decades many important contributions have come out of other groups in several institutes of the Department of Physics at ETH. These contributions range, to provide but a few examples, from fundamental works in mathematical physics [8–11], to proposals for novel types of material [12] and techniques relevant to the area of quantum technologies [13, 14], to experimental observations of intriguing topological effects both in natural [15, 16] and artificial structures [17].

Eugene Wigner delivered his lecture The Unreasonable Effectiveness of Mathematics in the Natural Sciences in 1959. He ended on a ‘cheerful note’: “The miracle of the appropriateness of the language of mathematics for the formulation of the laws of physics is a wonderful gift which we neither understand nor deserve. We should be grateful for it and hope that it will remain valid in future research and that it will extend, for better or for worse, to our pleasure, even though perhaps also to our bafflement, to wide branches of learning.” It seems fair to say that the branch of topology has delivered this sort of pleasure and bafflement — and keeps doing it.

Author: external page Andreas Trabesinger